Properties of Spiral Galaxies 

Luminosity Profiles

Unlike stars, galaxies are not point sources; their light is distributed over a patch of sky with a given angular size. So we describe their light distribution in surface brightness, which is flux per unit angular area. And just as flux can be measured in magnitudes, surface brightness can be measured in magnitudes per square arcsecond. In other words, if magnitudes work like this:

m = -2.5log(f) + c

surface brightnesses work like this:

mu = -2.5log(f/area) + c

And we can see the relationship between surface brightness and total magnitude by completing the math.

mu = -2.5log(f) + 2.5log(area) + c

mu = m + 2.5log(area)


As with the Milky Way, the surface brightness (flux per unit area) of spiral disks is described by an exponential law:

Or, if we convert this to magnitudes per square arcsecond:


Note that while the observed brightnesses and sizes of galaxies drop at larger distances, surface brightness does not change. Look at a patch of the galaxy of fixed angular size, and imagine moving the galaxy to larger distance.

The surface brightness of a galaxy is set by the density of stars inside it -- it is an intrinsic property of the galaxy,.


So we can measure the surface brightness of spiral galaxies and learn immediately the luminosity density -- the density of starlight in Lsun/pc2 -- inside the galaxy without knowing the distance. Very useful! In the B filter, mu = 27 mag/arcsec2 corresponds to 1 Lsun/pc2. A galaxy with a surface brightness of muB = 22 mag/arcsec2 has a luminosity density of 100 Lsun/pc2.


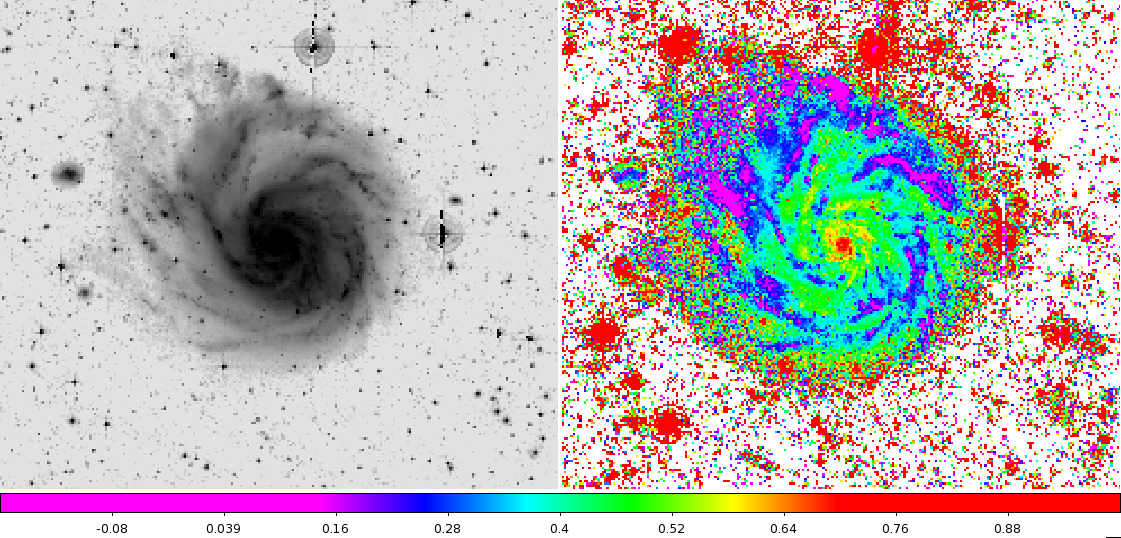
Galaxies show a wide range in central surface brightnesses; there is no preferred central surface brightness. On the left is M101, a high surface brightness galaxy; on the right is Malin 1, a low surface brightness galaxy.
 

M101
Malin 1


Colors and color gradients


The colors of spiral galaxies are affected by stellar populations -- the mix of young and old stars -- as well as dust. We saw that spiral galaxies are bluer than ellipticals, and that their colors also show a systematic trend with Hubble type: "Early" type spirals (Sa) are redder than "late" type spirals (Sc).





But in general, spirals also tend to show color gradients internally, where the outskirts are bluer than the inner regions. Example: M101 (from Mihos et al 2013):




And quantitative profiles of other disks from de Jong (1996):




So the outskirts of disk galaxies tend to be younger (and probably also less dusty) than the inner regions. This has led to the concept of "inside out" galaxy formation models, where the inner regions of galaxies form most of their stars early and the outer regions form stars more gradually over time.


How long can galaxies sustain star formation and keep building up their stellar mass? Typical star formation rates in big spirals are a few Msun/yr, and these galaxies typically have a few x 109 Msun of interstellar gas. So their gas depletion timescales are thus a few billion years, give or take, unless they can accrete gas from their surroundings.....


Kinematics of Spirals

 
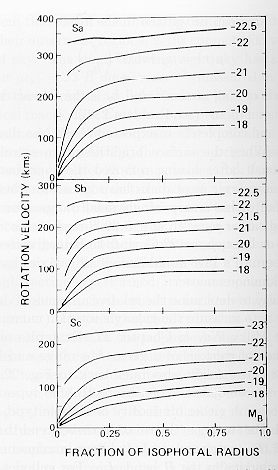
Spiral galaxies typically show flat rotation curves. Dark Matter!
 

The luminosity of a spiral galaxy correlates with its rotation velocity: the Tully-Fisher Relationship

or
or, in magnitudes

   
 
First, remember what determines the circular velocity:


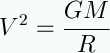so that


we don't know the mass of a galaxy, but we know its luminosity, so let's make up a quantity called the total mass-to-light ratio (which includes everything: stars, gas, dark matter):


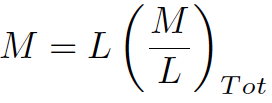now remember that surface brightness is luminosity over area:


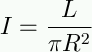or, solving for R:


OK. Now, mass is mass:


so equate our two mass expressions:


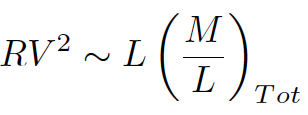substitute in for R:


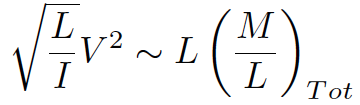and solve for L:

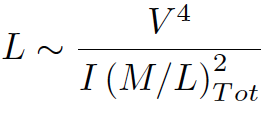Whew! So Tully-Fisher works if surface brightness times total (not stellar) mass-to-light-ratio squared is constant. In other words, the stars and the dark matter are somehow linked.

Why would that be true?
We don't understand it, but it seems to work!
But this tells us something fundamental about how galaxies formed. Any model for galaxy formation must explain the Tully-Fisher relationship.

OK, so let's look at the Tully-Fisher relationship for nearby galaxies using different wavelengths:

 
B (Blue) Tully Fisher
R (Red) Tully Fisher
H (Infrared) Tully Fisher

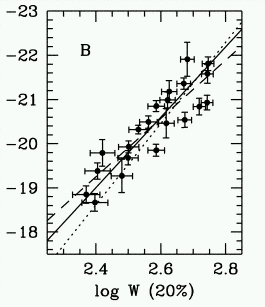X-axis: ~2Vcirc
Y-axis: absolute B magnitude

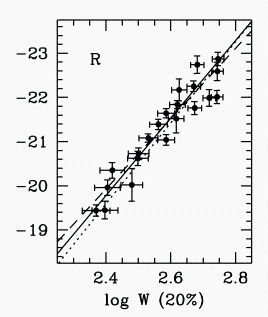X-axis: ~2Vcirc
Y-axis: absolute R magnitude

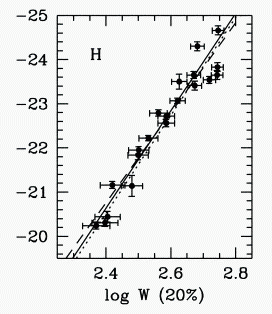X-axis: ~2Vcirc
Y-axis: absolute H magnitude
  • slope: -8.0
  • alpha: 3.2
  • scatter: 0.25 mag
  • slope: -8.8
  • slope: 3.5
  • scatter: 0.25 mag
  • slope: -11.0
  • alpha: 4.4
  • scatter: 0.19 mag

Question: Why would the relationship change depending on what wavelength you look at?


The Baryonic Tully-Fisher Relationship

(Figures from McGaugh 2005)

Let's look at a different version of the classic Tully-Fisher relationship: Blue luminosity versus circular speed for a sample of spiral galaxies.

We see the linear relationship, with a decent bit of scatter.

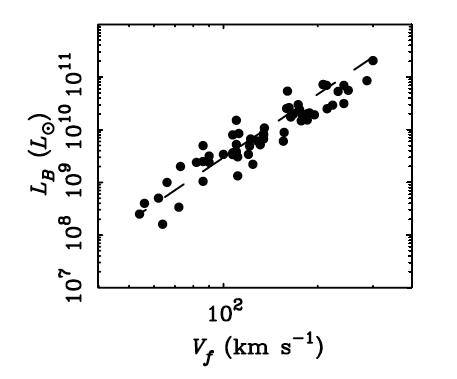Blue luminosity (LB) versus circular speed (Vf)
Now let's use the colors and luminosities of the galaxies, along with stellar population models, to work out the mass of all the stars in each galaxy. If we plot that on a TF-like diagram, the scatter is much less for massive galaxies, but the low mass galaxies don't fit.

But there's more stuff than just stars in a galaxy -- we haven't accounted for gas. Low mass spirals are preferentially more gas-rich, so we are missing a lot of their mass.

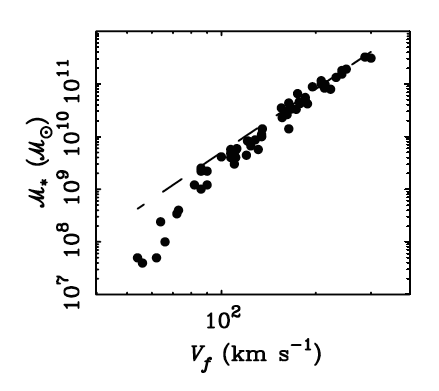Stellar disk mass (M*) versus circular speed (Vf)
If we define the total baryonic mass of the galaxy by adding both stars and gas together, we get any extremely tight relationship over orders of magnitude in mass!

There is a basic, fundamental relationship between the amount of normal (baryonic) mass in a spiral galaxy and the speed at which they rotate.

This is a huge constraint on models of dark matter and galaxy formation.

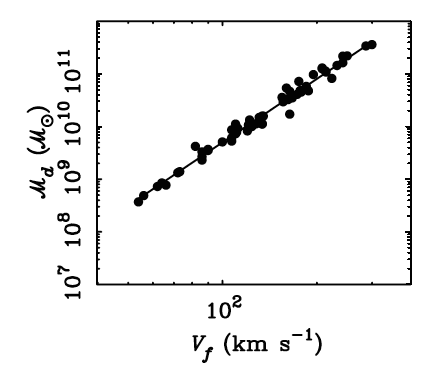Baryonic disk mass (Md) versus circular speed (Vf)