Galaxies: Colors, Star Formation, Stellar Population, and Mass-to-Light Ratios
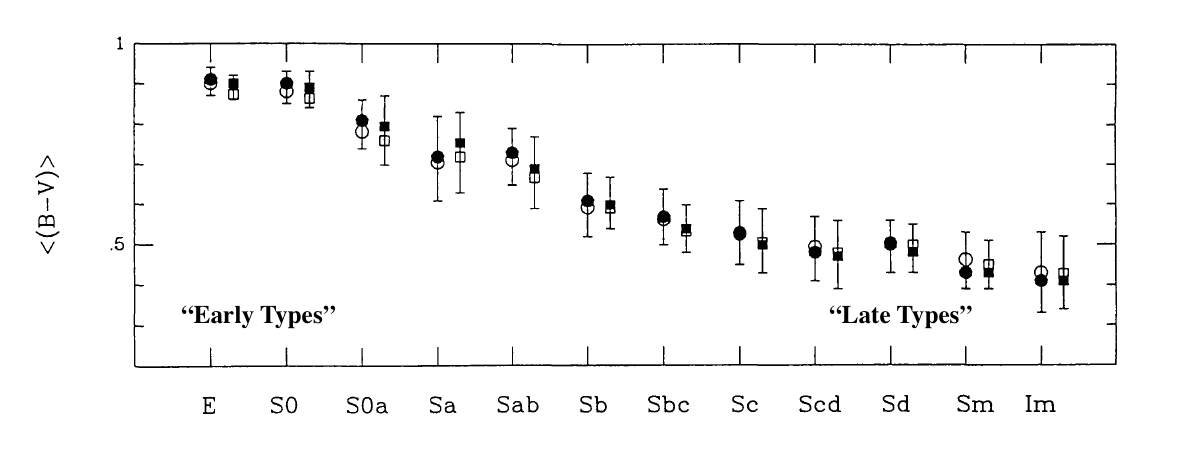
Along the Hubble sequence we find a trend of color with
galaxy type. E and S0 galaxies have red colors, and the colors get
progressively bluer for later galaxy types:
When we look at galaxies, we can only resolve individual stars in the very closest of galaxies
(Andromeda
and a few others). We generally study stellar populations of galaxies
using
integrated
colors or spectra. We
can use stellar population synthesis modeling to study how the spectra
and colors of a population of stars evolve with time, under different
input star formation histories. For example, an evolving population of
stars formed in a single burst of star formation looks like this:
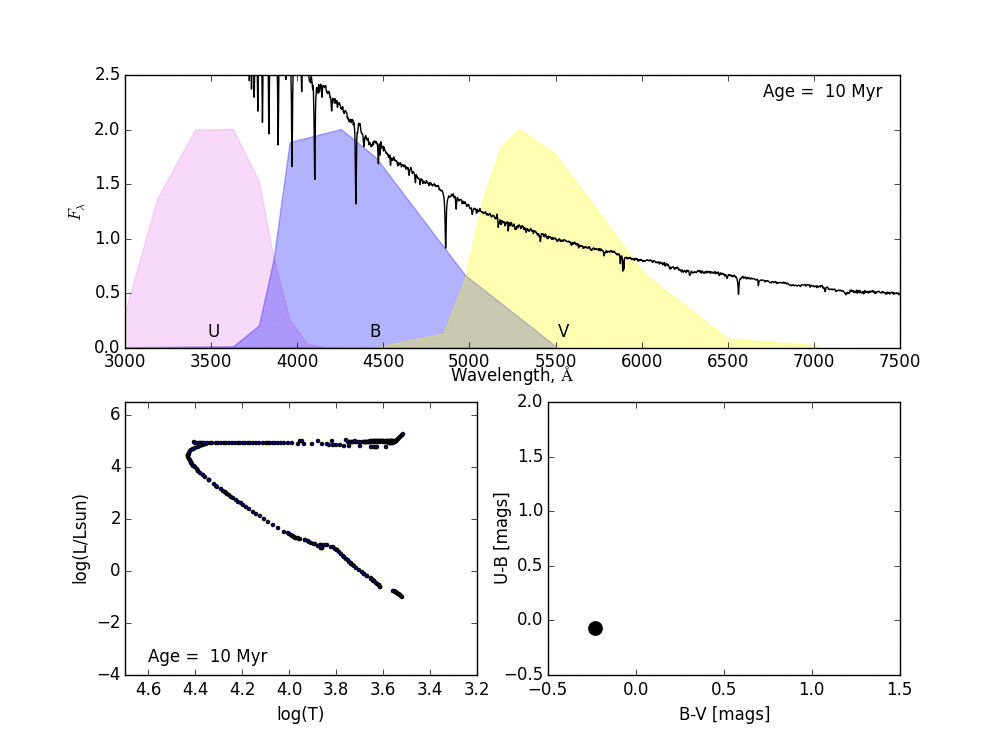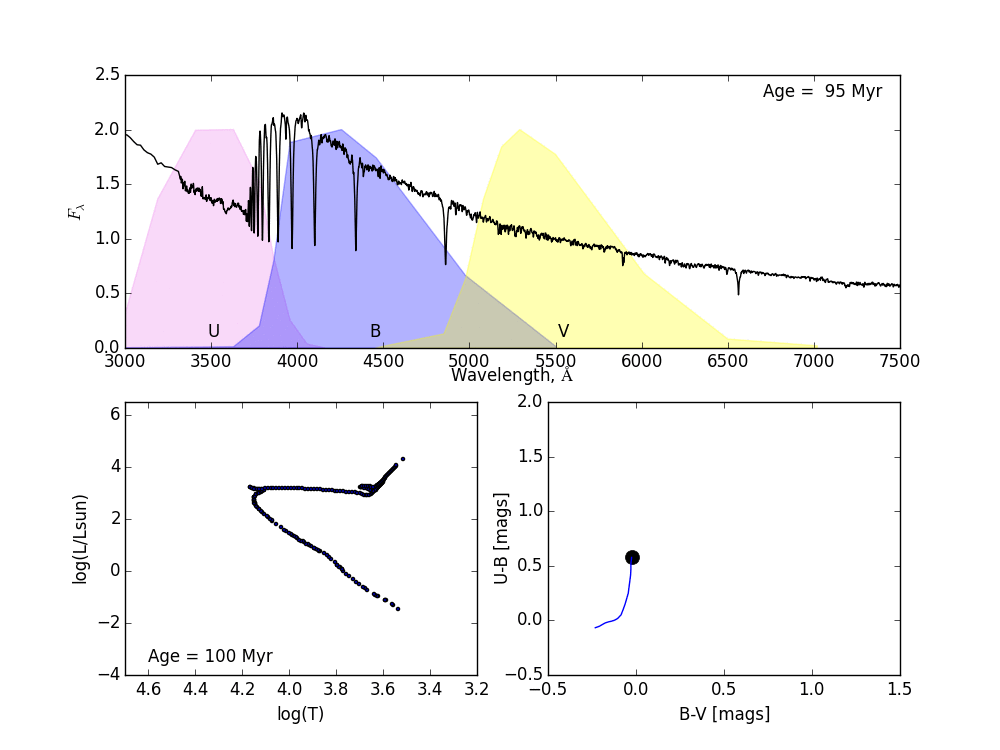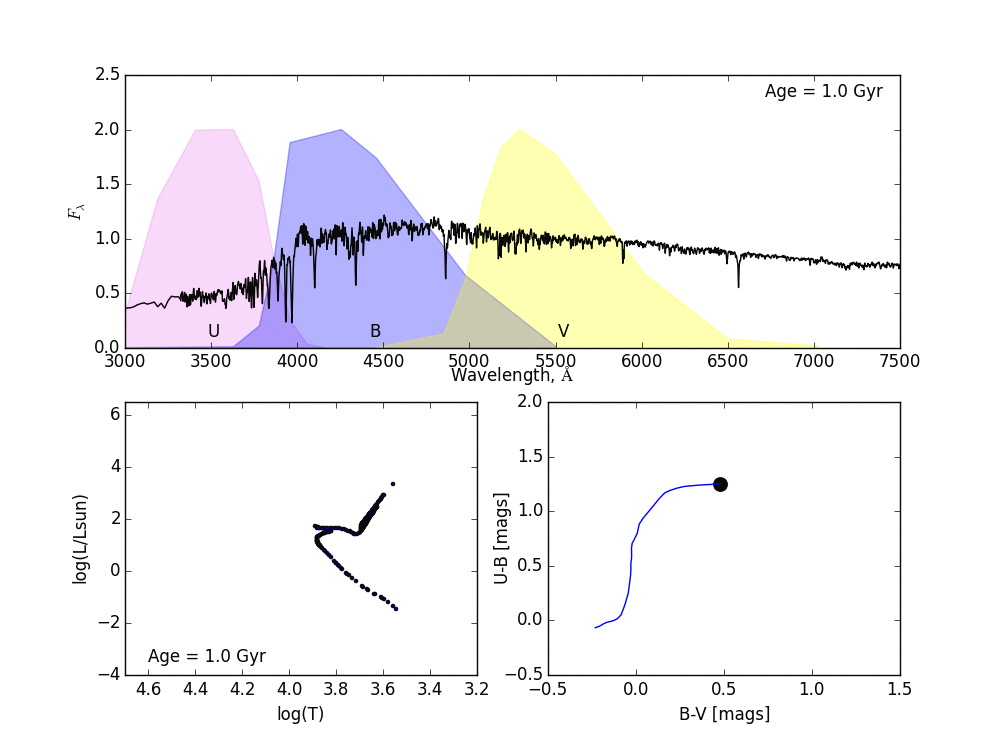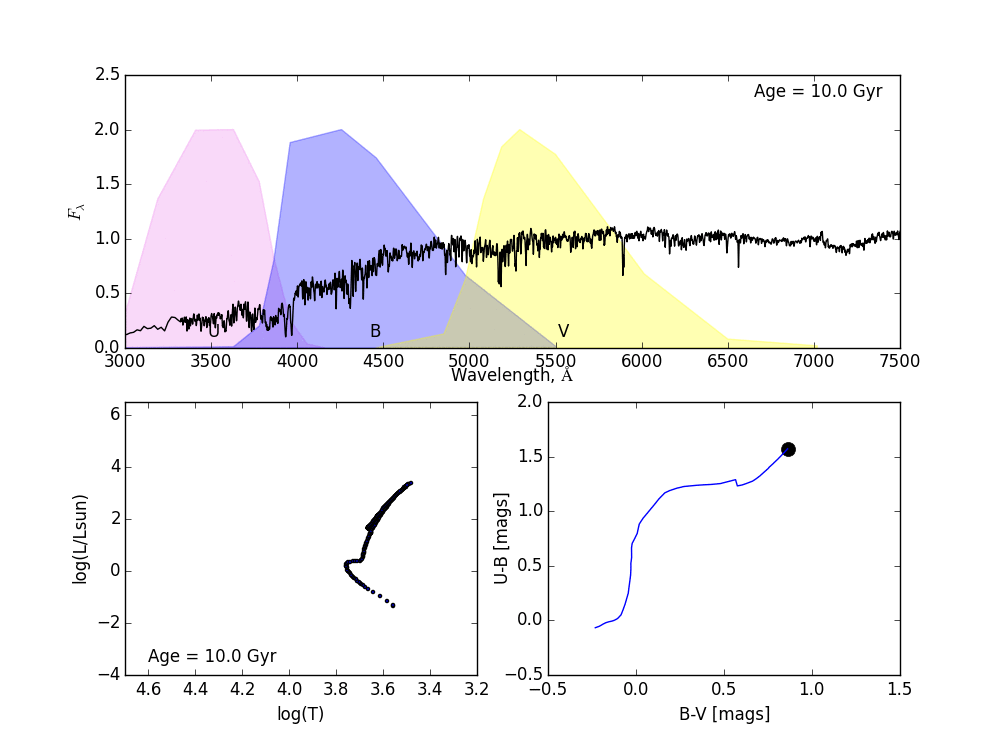
One very simple parameterization of star formation
history would be an exponentially declining star formation rate, with
differing e-folding timescales: SFR(t) ~ e-(t/tau) where tau
is left as a free parameter. (These are, not surprisingly, called "tau
models"). Imagine galaxies with four different star formation histories
(different taus) and watch how evolve differently in color:
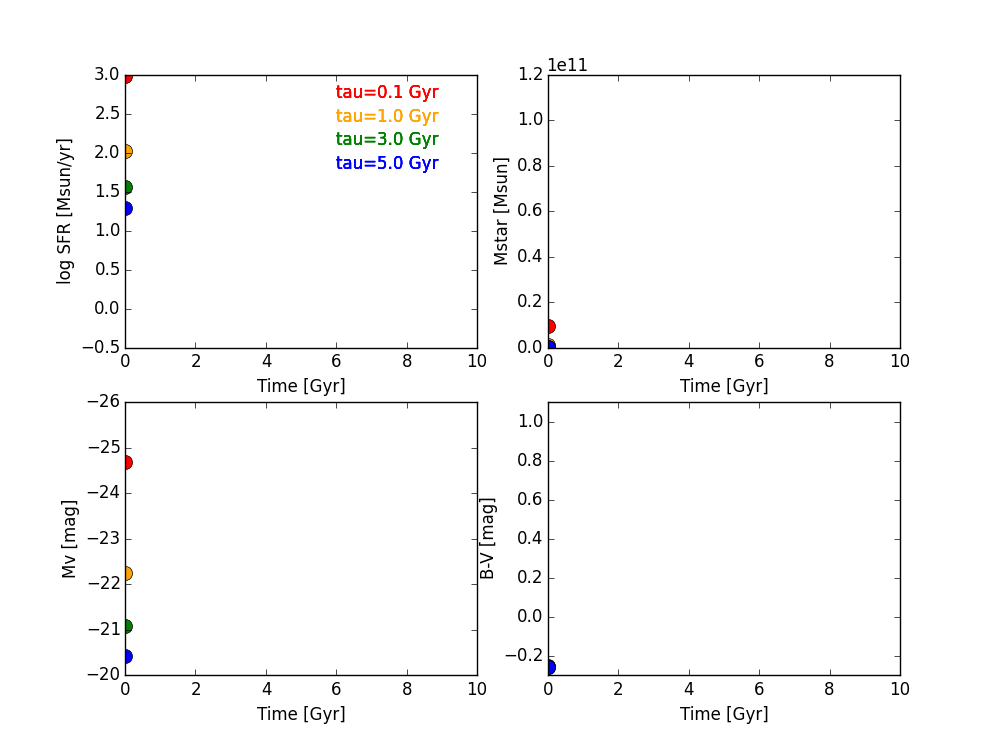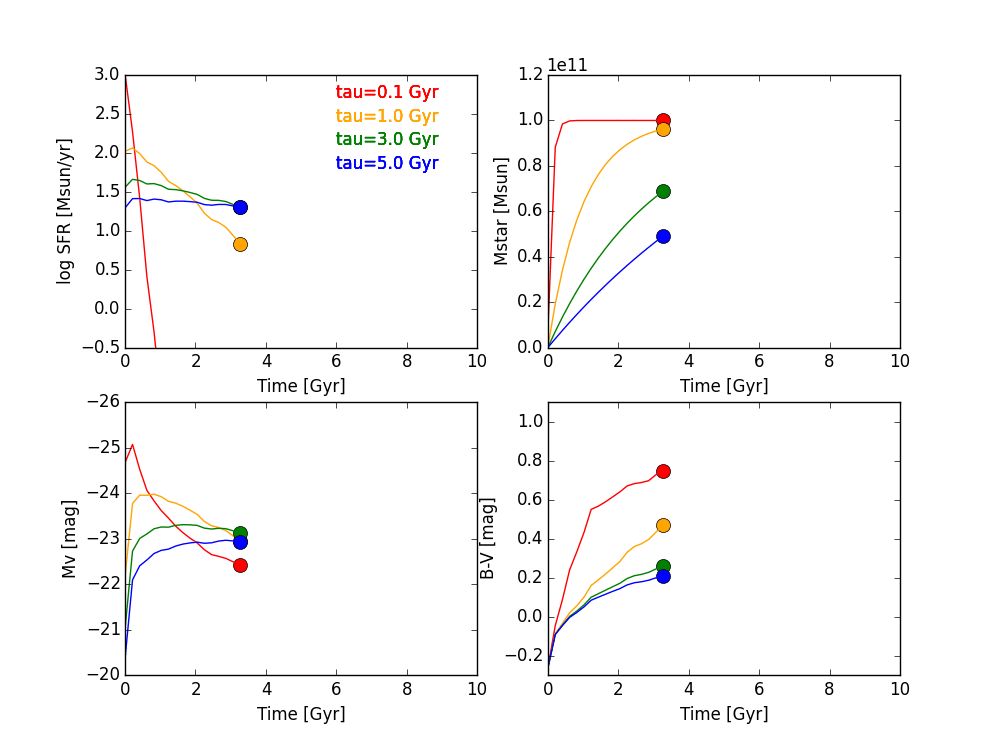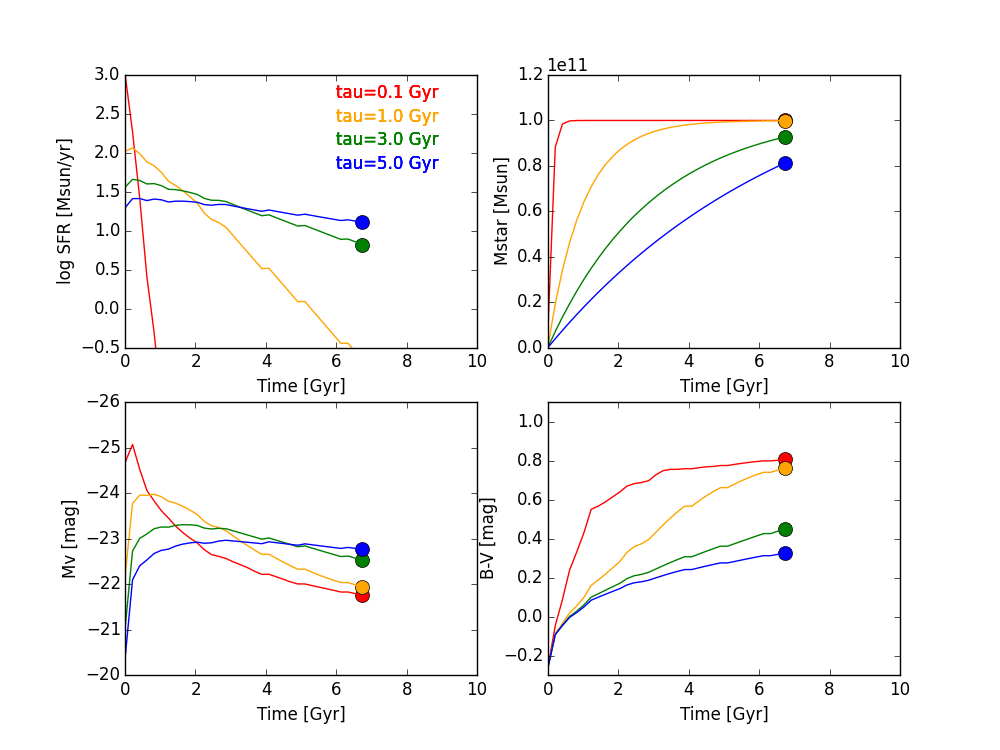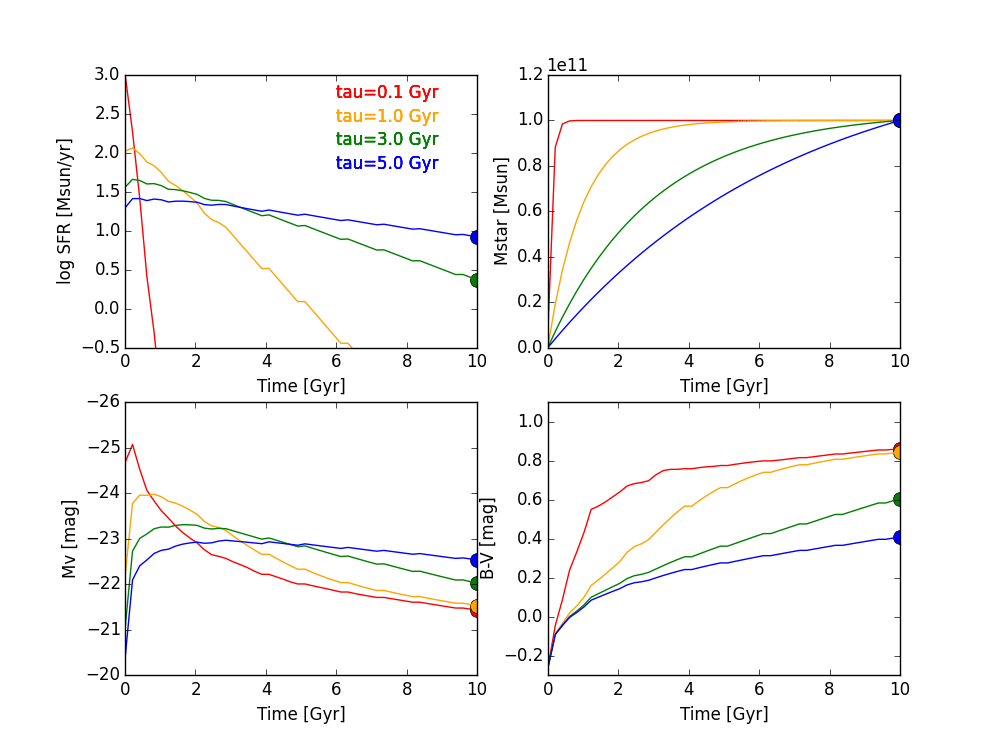
So red galaxies have stars which, on average, are generally older, while bluer galaxies have younger stars.
(Thanks to Ken Duncan for his population synthesis software that helped make these plots!)
Stellar Mass-to-Light Ratios
If we understand the properties of the stellar populations we
are observing, we can use their total luminosity to infer the amount of
mass in stars by invoking the stellar mass-to-light ratio, written (M/L)*.
This quantity has units of Msun/Lsun, so the Sun has a (M/L)* = 1 Msun / 1 Lsun = 1 Msun/Lsun.
An A-type main sequence star might have a luminosity of 12 Lsun and a mass of 2 Msun, so it would have a (M/L)* = 2 Msun / 12 Lsun = 0.17 Msun/Lsun.
A faint K-type main sequence star would have L = 0.3 Lsun and mass = 0.75, so (M/L)* = 0.75 Msun / 0.3 Lsun = 2.5 Msun/Lsun.
To get the (M/L)* for a population of stars,
you sum up all their light to get the total luminosity, sum up all
their mass to get the total mass, and then divide mass by light. Since
we almost never see the individual stars in a galaxy (most galaxies are
too far away to see individual stars), we usually have to infer the
stellar population by modeling the integrated colors or spectrum of the
galaxy. But then we can take the observed luminosity and the inferred (M/L)*
to estimate the total mass in stars in a galaxy -- its "stellar mass"
(which is very different from its total mass, which would include gas
and dark matter as well).