Astr 221 Homework #1 - due Sep 8th

1. Incoming!

It's the year 2020. You discover a new comet as it crosses the orbit of Jupiter heading in towards the Sun. At this point, your observations show that it is moving at a speed of 18 km/s. You further calculate that the perihelion distance of the comet will be exactly 1 AU! Excited by the fact that it will come so close to the Earth's orbit, you think of all the fame you will get for discovering this comet, which you decide to name Comet Mihos in honor of your undergraduate astronomy professor who started you on your career in astronomy. As you begin writing your press release, old Dr. Blowhard comes up to you and says "I discovered that comet the last time it passed by Earth in 1973." Your observations also indicate that the comet is approximately 5 kilometers across; assuming it is roughly spherical, and knowing that comets have an average density of about 1.5 g/cm3, what do you estimate the mass of the comet to be?

You realize that the potential exists for a collision between this comet and the Earth, so you start to worry about how much damage would be done by an impact. If the comet hits the Earth, how much kinetic energy will be released? (Think carefully about the relative velocity between the Earth and the comet) If a hydrogen bomb releases about 15 megatons of energy, how many H-bombs is this impact equivalent to?

Do you want to be around if Comet Mihos hits?

2. Mission to Mars

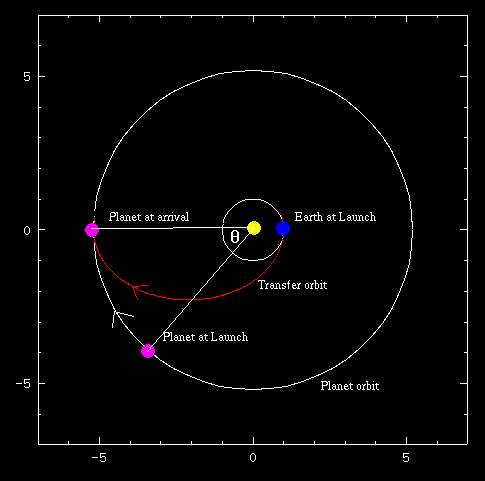
In this problem, we will calculate the parameters of a Hohmann transfer orbit which will take us from Earth to Mars. A Hohmann orbit is an orbit which goes from one planet to another using the least amount of energy. It is an elliptical orbit whose perihelion is at the Earth's orbit, and whose aphelion just reaches the orbit of the other planet (see figure to the right). 

The trick, of course, is that the other planet has to be at the right spot to rendezvous with the spacecraft!

So you need to calculate three things:

  • a, the semimajor axis of the transfer orbit to Mars
  • e, the eccentricity of the transfer orbit to Mars
  • theta, the angle between Mars' position when the probe is launched and Mars' position at rendezvous.
Calculate these three numbers, then use the  "Mission to Mars" JavaLab applet to see if your solution works.

(For more fun with transfer orbits, you can also play with the Grand Tour applet.)

3.  I want my MTV!

You want to put up a communications satellite so that it appears fixed in the sky (so you don't need to keep repointing your satellite dish!).  What is the  semi-major axis (a), eccentricity (e), and period (P) of the orbit it needs to be on? How is that orbit oriented with respect to the Earth's equator?

4. Weighing Saturn

Use the orbital data for Titan (Appendix D, Table D.2 of your text) to calculate the mass of Saturn.

5.  Units, units, units

Using astronomical units as the unit of length, years as time, and the mass of the Sun as the unit of mass, the value for k in Kepler's Third Law is 1.0. In these units, what is the value for Newton's constant, G?